Cover walks on a grid

James Tanton discusses with Sunil Singh, in the video interview below, the problem he thought about as a young child, that led – years later – to an epiphany and to a deeper understanding of mathematical thinking:

The thought that led James to a solution – a reason why some squares in a grid cannot be the origin of a walk through adjacent squares that reaches each square exactly once – came from coloring squares alternately one of two colors:

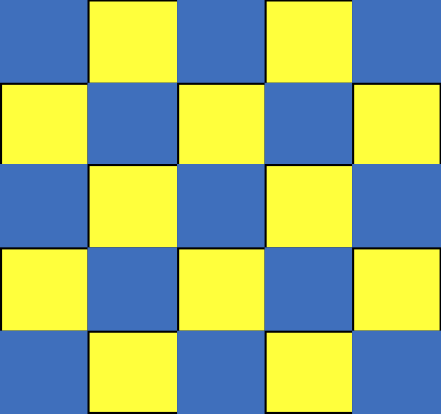
In the grid colored as shown, there are 13 blue squares and 12 yellow squares. Now a moment’s thought will reveal that starting from a yellow square there is no walk through adjacent squares – which, as you can see, flips alternately between the two colors –  that reaches each square exactly once.

BY BY BY BY BY BY BY BY BY BY BY BY B  (doesn’t rule out such a walk)

YB YB YB YB YB YB YB YB YB YB YB YB Y (proves there’s no such walk)